Resolvemos problemas de matemáticas respondiendo a preguntas sobre tus deberes de álgebra, geometría, trigonometría, cálculo diferencial y estadísticas con explicaciones paso a paso, como un tutor de matemáticasView MATLAB Command Show volumetric data along slice planes that are orthogonal to each axis Create slice planes through the volume defined by , where x, y, and z range from 2,2 Create slice planes orthogonal to the x axis at the values 12, 08, and 2 and orthogonal to the z axis at the value 0 Do not create any slice planes that are26/7/ Straight line graphs y = mx c is an important reallife equation The gradient, m, represents rate of change (eg, cost per concert ticket) and
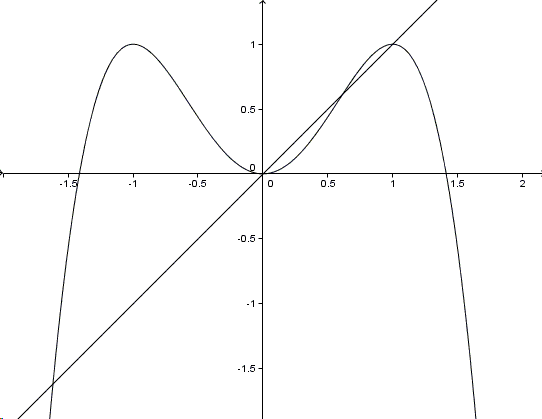
Graphing Equations Is Useful Ii
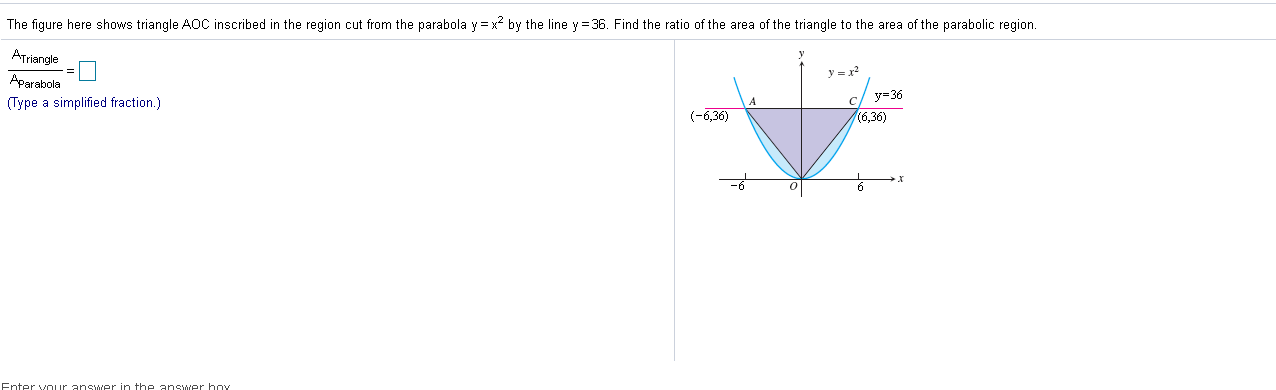
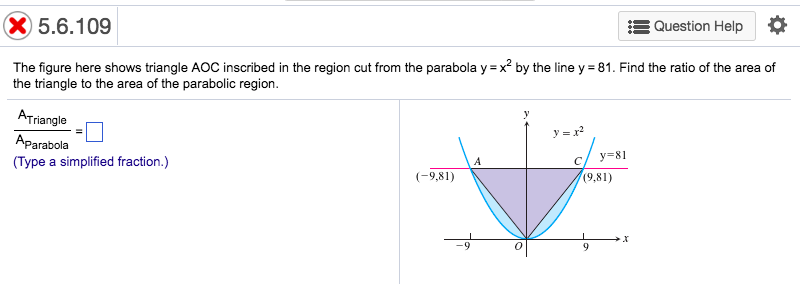
The line x+y=2 cuts the parabola
The line x+y=2 cuts the parabola-Solution Figure 156 displays the volume beneath the surface By Fubini's Theorem, Reversing the order of integration gives the same answer EXAMPLE 2 Find the volume of the region bounded above by the ellipitical paraboloid and below by the rectangle Solution The surface and volume are shown in Figure 157 The volume is given by the12/4/21 To set the Xaxis values, from the Fields pane, select Time > FiscalMonth To set the Yaxis values, from the Fields pane, select Sales > Last Year Sales and Sales > This Year Sales > Value Now you can customize your Xaxis Power BI gives you almost limitless options for formatting your visualization




Draw The Graph Of Each Of Equations Given Below Also Find The Coordinates Of The Points Where The Graph Cuts The Coordinate Axes 3x 2y 6 0
Click here👆to get an answer to your question ️ Find the point on the curve y = x^2 2x 3 , where the tangent is parallel to x axis26/7/ Differentiate algebraic and trigonometric equations, rate of change, stationary points, nature, curve sketching, and equation of tangent in Higher MathsIn mathematics, a Fourier series (/ ˈ f ʊr i eɪ,i ər /) is a periodic function composed of harmonically related sinusoids, combined by a weighted summationWith appropriate weights, one cycle (or period) of the summation can be made to approximate an arbitrary function in that interval (or the entire function if it too is periodic)As such, the summation is a synthesis of another function
The parallel wires are labeled a, b, and, c, and the angles are labeled with numbers The measure of one angle is 130° Which statement is true regarding the 130° angle and angle 3?Free parallel line calculator find the equation of a parallel line stepbystepClick here👆to get an answer to your question ️ The curves x^2 y^2 = 5 and x^2/18 y^2/8 = 1 cut each other at the common point at an angle
They are sameside interior angles, so angle 3 measures 50° They are3/8/17 We compute the partial derivative of a function of two or more variables by differentiating wrt one variable, whilst the other variables are treated as constant Thus The First Derivatives are f x = ∂f ∂x = 3y −3x2 f y = ∂f ∂y = 3x − 6y The Second Derivatives are f xx = ∂2f ∂x2 = −6x f yy = ∂2f ∂y2 = − 6Problem 1 (15 points) Find the absolute maximum and minimum values of f(x;y) = exy on the domain 2x2 y2 1 Solution We rst check for critical points on the interior of the domain using the



1



What Is The Distance Between Two Points If X Y 1 Cuts Circle X 2 Y 2 1 At Two Points Quora
Answer As we can see in the gure, the line y= 2x 7 lies above the parabola y= x2 1 in the region we care about26/5/ In this section we will start evaluating double integrals over general regions, ie regions that aren't rectangles We will illustrate how a double integral of a function can be interpreted as the net volume of the solid between the surface given by the function and the xyplaneSet y y equal to the new right side y = x 2 y = x 2 y = x 2 y = x 2 Use the vertex form, y = a ( x − h) 2 k y = a ( x h) 2 k, to determine the values of a a, h h, and k k a = 1 a = 1 h = 0 h = 0 k = 0 k = 0 Since the value of a a is positive, the parabola opens up Opens Up




11 A Digraph With 2 Cut X Y Neither Of The Bridges Of This 2 Cut Download Scientific Diagram




Makita 10 Pack 4 5 Cut Off Wheels For Grinders Aggressive Cutting For Metal Stainless Steel Inox 4 1 2 X 045 X 7 8 Inch Amazon Com
Y= x 2 z cut o by the plane y= 25 Solution Surface lies above the disk x 2 z in the xzplane A(S) = Z Z D p f2 x f z 2dA= Z Z p 4x2 4y2 1da Converting to polar coords get Z 2ˇ 0 Z 5 0 p 4r2 1rdrd = ˇ=8(101 p 101 1) Section 167 2Search the world's information, including webpages, images, videos and more Google has many special features to help you find exactly what you're looking forSolid enclosed by the parabolic cylinders y = x2 and y =8 x2 and the planes z =0and 3x4y z = 9 Do not calculate the double integral Z Z dydx 11 Recall that the volume of a sphere of radius r>0is(4/3)⇡r3 In the following, you should think in terms of the definition of a double integral




The Figure Here Shows Triangle Aoc Inscribed In The Region Cut From The Parabola Y X 2 By The Line Brainly Com
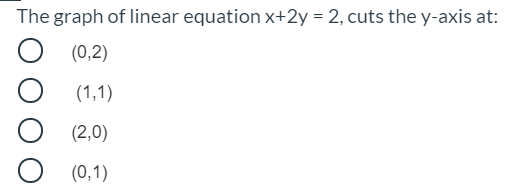



Answered The Graph Of Linear Equation X 2y 2 Bartleby
Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreIf the curves a yx^2=7a n dx^3=y cut orthogonally at (1,1) , then find the value adot Apne doubts clear karein ab Whatsapp par bhi Try it now Find the surface area of the paraboloid z=x^2y^2 cut by z=2 1 answer below » Find the surface area of the paraboloid z=x^2y^2 cut by z=2 1212 AM 1 Approved Answer (x,y) = x 2 y 2 over the region in the xy plane bounded by 2




The Graph Of Linear Equation X 2y 2 Cuts The Y Axis At O 2 0 O 0 2 O 1 1 0 1 Brainly In




How Do You Write The Definite Integral To Find The Smaller Area Cut From The Circle X 2 Y 2 25 By The Line X 3 Socratic
Assignment 7 (MATH 215, Q1) 1 Find the area of the given surface (a) The part of the cone z = p x2 y2 below the plane z = 3 Solution The surface can be represented by the vector equationSOLUTIONS TO MAXIMUM/MINIMUM PROBLEMS SOLUTION 1 Let variables x and y represent two nonnegative numbers The sum of the two numbers is given to be 9 = x y , so that y = 9 x We wish to MAXIMIZE the PRODUCT P = x y2 However, before we differentiate the righthand side, we will write it as a function of x onlyTo find the answer, make a data table Data Table for y = x 2 And graph the points, connecting them with a smooth curve Graph of y = x 2 The shape of this graph is a parabola Note that the parabola does not have a constant




Linbo3 Saw Grade Wafer 128 Deg Y X Cut 4 Dia X1 0 Mm 2sp Lnsy101d10c2deg128



Sec 4 8 The X And Y Intercepts Ppt Download
Y x 2 2 1 1 4 4 Economics 3070 c U(x, y) = x2/3 y1/3 Since the indifference curves are bowed towards the origin, they do obey the assumption of diminishing MRS y x 8 8 1 1 512 512 Economics 3070 d U(x, y) =min(2X, 3Y) This is an example of perfect complements1/7/ Here we can clearly see that the quadratic function y = x^{2} does not cut the xaxis But the graph of the quadratic function y = x^{2} touches the xaxis at point C (0,0) Therefore the zero of the quadratic function y = x^{2} is x = 0 Now you may think that y = x^{2} has one zero which is x = 0 and we know that a quadratic function has 2 zeros14/4/21 Ex 63, 23 Prove that the curves 𝑥=𝑦2 & 𝑥𝑦=𝑘 cut at right angles if 8𝑘2 = 1We need to show that the curves cut at right angles Two Curve intersect at right angle if the tangents to the curves at the point of intersection are perpendicular to each other First we Calculate the point of inters




Diablo 4 1 2 In Thin Kerf Metal Cut Off Disc For X Lock And 7 8 In Arbor Angle Grinders Dbxf The Home Depot



Graphs Of Surfaces Z F X Y Contour Curves Continuity And Limits
Stack Exchange network consists of 177 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack ExchangeFind the area of the finite part of the paraboloid y = x^2 z^2 cut off by the plane y = 16 (Hint Project the surface onto the xzplane) View AnswerTextbook solution for Calculus (MindTap Course List) 8th Edition James Stewart Chapter 155 Problem 23E We have stepbystep solutions for your textbooks written by Bartleby experts!
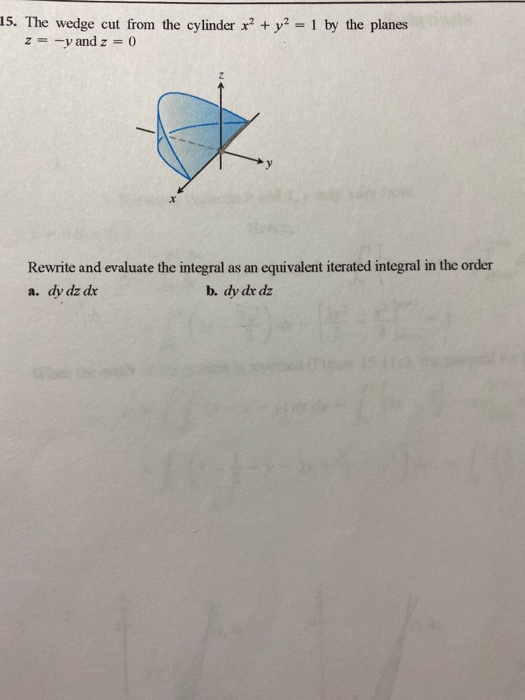



15 The Wedge Cut From The Cylinder X2 Y2 1 By Chegg Com




Evaluate The Volume Of The Solid Bounded By Z 8 X 2 Y 2 Z X 2 Y 2 X 1 Y Sqrt 3 X Y 0 Mathematics Stack Exchange
Math 2260 Exam #1 Practice Problem Solutions 1What is the area bounded by the curves y= x2 1 and y= 2x 7?Over the region D = {(x,y) x2 y2 8} As before, we will find the critical points of f over DThen,we'llrestrictf to the boundary of D and find all extreme values It is in this second step that we will use Lagrange multipliers The region D is a circle of radius 2 p 2Find the area bounded by the curve y = x2x4, the xaxis and the ordinates x = 1 and x = 3 Solution If we set y = 0 we obtain the quadratic equation x2 x 4 = 0, and for this quadratic b2 − 4ac = 1− 16 = −15 so that there are no real roots This means that the curve does not cross the x



Algebra Parabola Transformations Of Y X 2 Graphs Match Up 2 Teaching Resources



Find The Volume Enclosed By The Cylinder Y 2 X And Y X 2 Cut Off By The Planes Z 0 X Y Z 2 Applied Mathematics 2 Shaalaa Com
Get answer A The curve y=x^2,6y=7x^3 cut orthogonally at (1,1) R Two curve cut each other orthogonally at their point of intersection P iff m_1m_2=1 where m_1,m_2 are the gradiants of the two curves at PIf the graph of y = x 2 X2 cuts the xaxis, then y = on studyassistantphcomMath V12 Calculus IV, Section 004, Spring 07 Solutions to Practice Final Exam Problem 1 Consider the integral Z 2 1 Z x2 x 12x dy dx Z 4 2 Z 4 x 12x



Q Tbn And9gcqmy0k2kb Jgbw2scivj1dzbk6nkx5c Kl4hf Umrluyemtwch3 Usqp Cau




Find The Surface Area Cut From The Plane 2x Y Z 0 By The Cylinder X 2 Y 2 4 Study Com
The earliest known work on conic sections was by Menaechmus in the 4th century BC He discovered a way to solve the problem of doubling the cube using parabolas (The solution, however, does not meet the requirements of compassandstraightedge construction)The area enclosed by a parabola and a line segment, the socalled "parabola segment", was computed byWolframAlpha brings expertlevel knowledge and capabilities to the broadest possible range of people—spanning all professions and education levels23/9/11 13–86 A 2kg particle travels along a horizontal smooth path defined by 1 t2 r = ¢ t3 2 ≤ m, u = ¢ ≤ rad, 4 4 where t is in seconds Determine the radial and transverse components of




Graphing Linear Inequalities Chilimath




Given A Lamina Cut By The Parabolas Y X2 And X Y2 Chegg Com
Graphing y = x 2 We have already discovered how to graph linear functions But what does the graph of y = x 2 look like?Math 9 Assignment 5 Solutions 3 8 Find the surface area of the paraboloid z = 4 x2 y2 that lies above the xyplane Solution For this problem polar coordinates are useful S = ZZ22/8/ Respuestax³ xy² x²y y³Explicación paso a paso(x y) • (x 2 y 2)= Multiplica cada termino del binomio con cada termino del otro factor(x y) • (x 2 y



Draw The Graph Of The Linear Equation 3x 4y 6 At What Points Does The Graph Cut The X Axis And The Y Axis Studyrankersonline




Find The Area Of The Finite Part Of The Paraboloid Y X 2 Z 2 Cut Off By The Plane Y 4 Hint Project The Surface Onto The Xz Plane Study Com
Academiaedu is a platform for academics to share research papers6/1/21 Online Question and Answer in Differential Calculus (Limits and Derivatives) Series Following is the list of multiple choice questions in this brand new series MCQ in Differential Calculus (Limits and Derivatives) PART 1 MCQ from Number 1 – 50 Answer key PART 1 PART 2 MCQ from Number 51 – 100 Answer key PART 2Chapter 5 Line and surface integrals Solutions Example 51 Find the work done by the force F(x,y) = x2i− xyj in moving a particle along the curve which runs from (1,0) to (0,1) along the unit circle and then from (0,1) to (0,0) along the yaxis (see
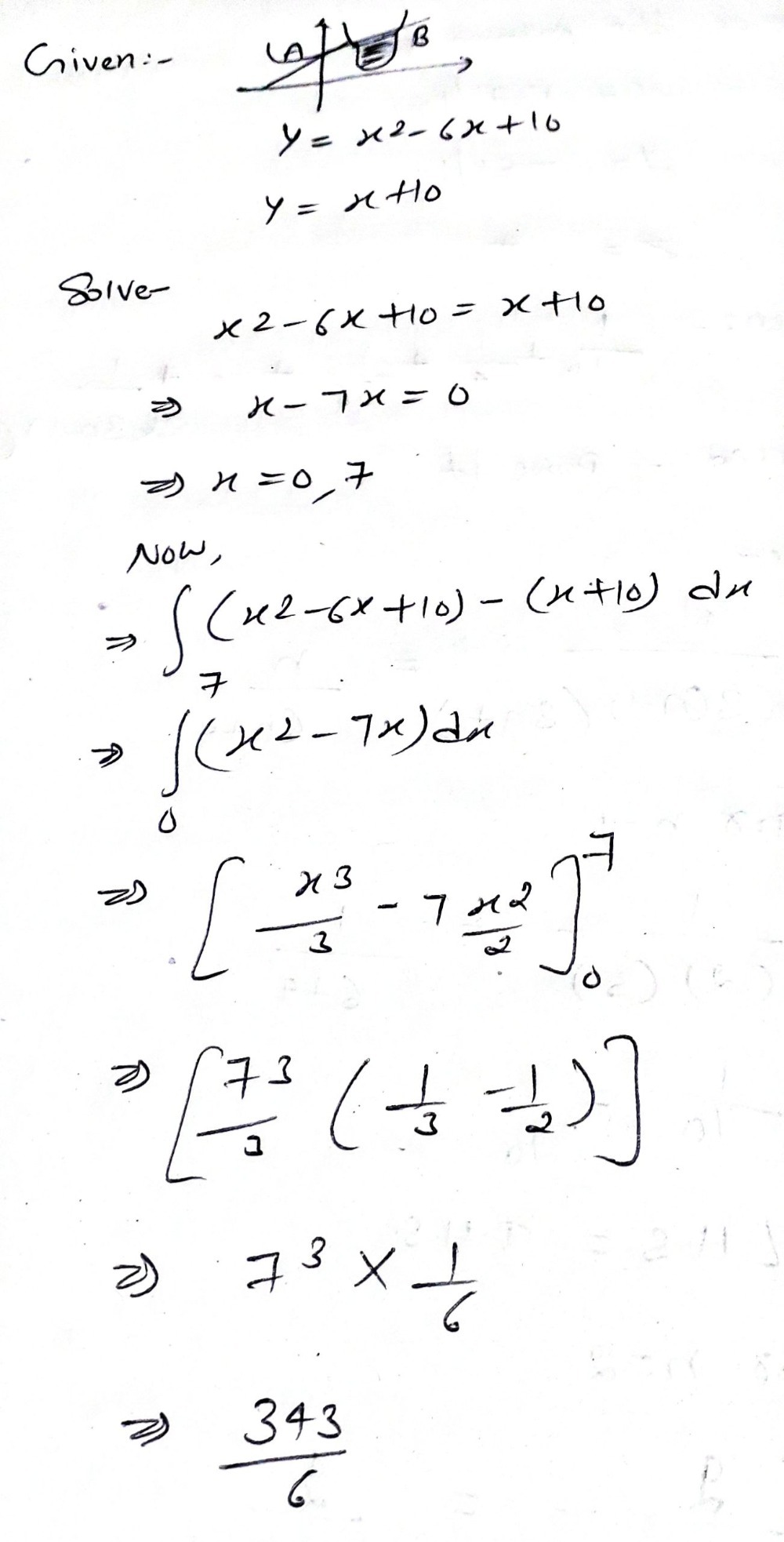



The Graph Of Y X2 6x 10 Cuts The Y Axis At A The Gauthmath




Angelo Cuts A Piece Of Wood For A Project The First Cut Is Shown And Can Be Represented By The Brainly Com
30/4/18 Find the equation of the tangent to the curve y(x – 2)(x – 3) – x 7 = 0 at the point where it cuts the xaxis asked in Mathematics by KumarManish ( 577k points) differential equations9 Find the area of the region bounded by the parabola y = x^2 and y= xarea of region bounded,area of a bounded region,area of the region bounded by the gr16/5/18 How do you calculate the arc length of the curve #y=x^2# from #x=0# to #x=4#?



Graphs Of Surfaces Z F X Y Contour Curves Continuity And Limits



The Figure Here Shows Triangle Aoc Inscribed In The Chegg Com
6 Chapter 6 Applications of the Integral 28 Figure 16 Figure for Problem 28 29 x = y2 — 5 x = 3 — y2 Figure 17 Figure for Problem 29 We have 2 − 2 3 − y2 − y2 −5 dy= 2 −2 8 −2y2 dy= 8y − 2 3 y3 − = 30 Figure 18 shows the graphs of x = y3 −26y 10 and x = 40 −6y2 − y3Match the equations with the curve and compute the area of the shaded regionCalculus Applications of Definite Integrals Determining the Length of a Curve 1 Answer Eric S Use the arc length formula Explanation #y=x^2# #y'=2x# Arc length is



16 The Graph Of Y X2 6x 10 Cuts The Y Axis At A Gauthmath




The Figure Here Shows Triangle Aoc Inscribed In The Chegg Com




Portion Of Asymptote Of Hyperbola X 2 A 2 Y 2 B 2 1 Between Centre And The Tangent At Vert Youtube




The Length Of The Chord Cut Off By Y 2x 1 From The Circle X2 Y2 Maths Three Dimensional Geometry Meritnation Com



Pinkmonkey Com Calculus Study Guide Section 8 5 Arc Length Rectification



5 6 109 Question Help The Figure Here Shows Chegg Com



What Is The Length Of The Arc Of The Parabola Y2 4ax Cut Off By The Line 3y 8x Quora




5 The Figure Here Shows Triangle Aoc Inscribed In Chegg Com



How To Find The Volume Of The Solid Generated By Revolving The Region Between The Parabola Math X Y 2 1 Math And The Line Math X 3 Math About The Line Math X 3 Math Quora




Draw The Graph Of Each Of Equations Given Below Also Find The Coordinates Of The Points Where The Graph Cuts The Coordinate Axes 3x 2y 6 0
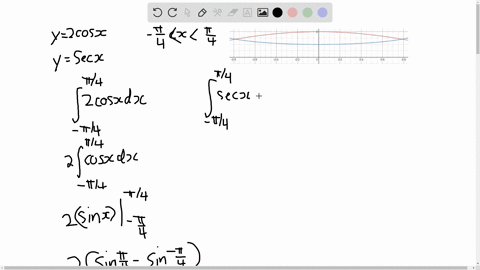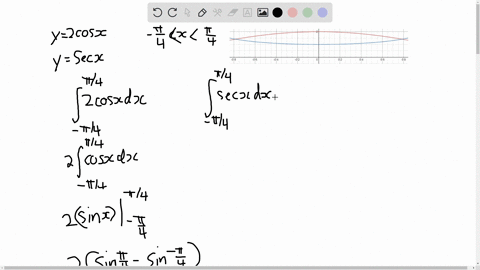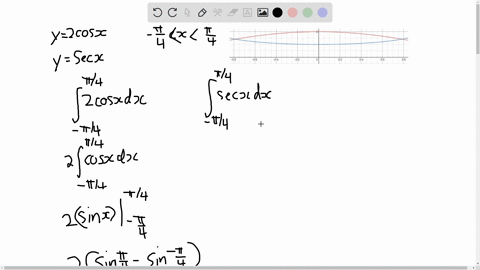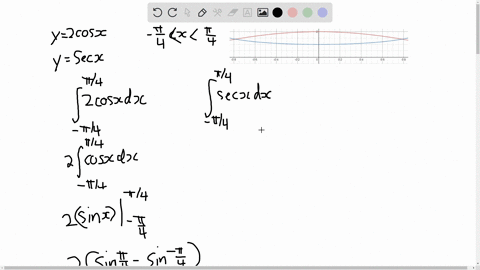



Solved Circular Sector Integrate F X Y Sqrt 4




The Graph Of The Linear Equation 2x Y 4 Cuts X A Xi S At A 2 0 B 2 0 Youtube




Graphing Equations Is Useful Ii



Find The Area Of The Segment Cut Off From The Parabola Y 2 2x By The Line Y 4x 1 Sarthaks Econnect Largest Online Education Community




Pin On My Tpt Store All Things Algebra




Find The Angle At Which The Curve Y 2x X 2cut The X Axis




14 3 Partial Differentiation




Quadratic Graphs Parabolas 1 To Draw A Quadratic



Stewart Calculus 8e Chapter 5 Plus Problems




Richard Cuts A Peice Of Wood For A Project The First Cut Is Shown And Can Be Represented By The Brainly Com




Draw The Graph Of The Equation 3x 2y 6 Find The Coordinates Of The Point Where The Graph Cuts The Y Axis



Hartleymath Triple Integrals




Find The Length Of The Chord Cut Off By Y 2x 1 From The Circle X 2 Y 2 2 Youtube
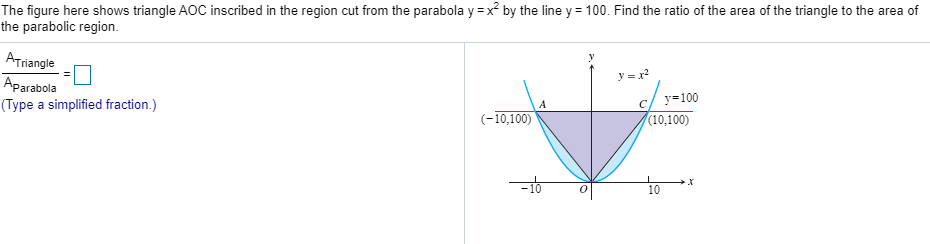



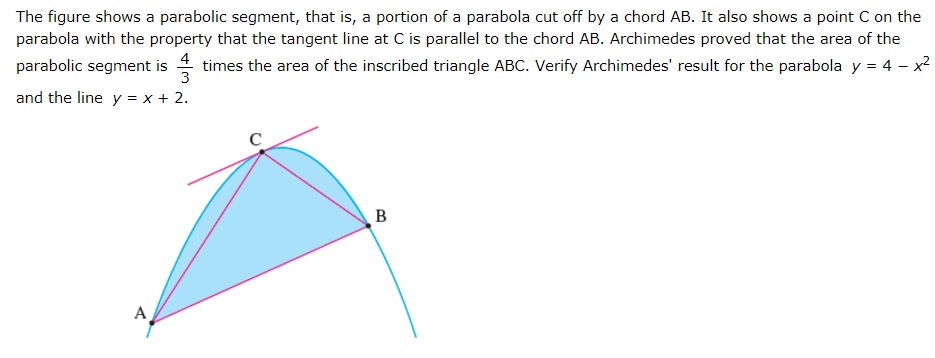
The Figure Here Shows Triangle Aoc Inscribed In The Chegg Com




View Question The Square With Vertices A A A A A A A A Is Cut By The Line Y X 2 Into Congruent Quadrilaterals The Perimeter Of O
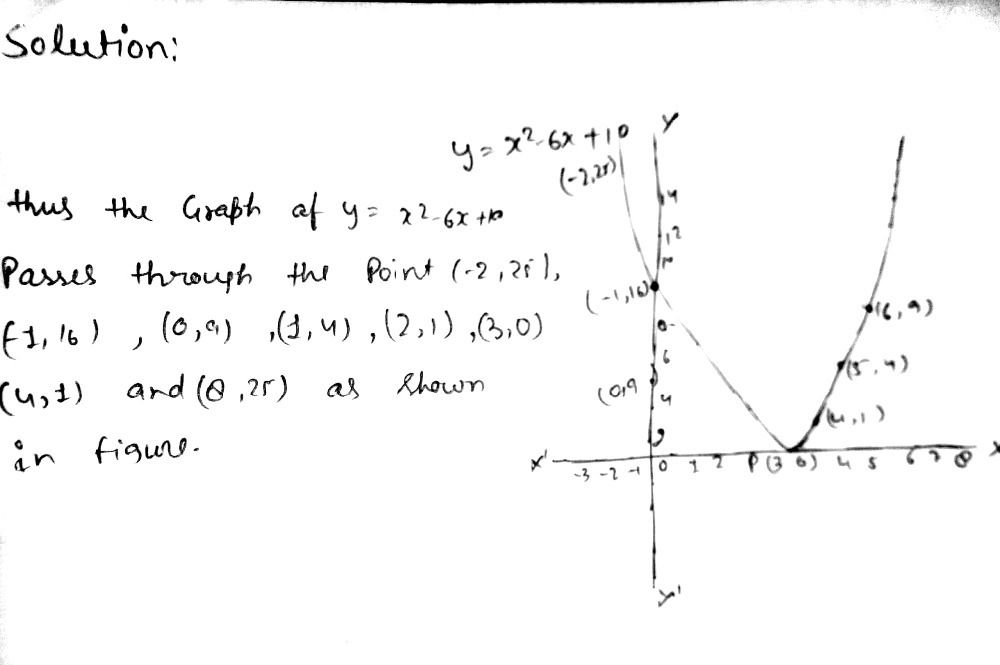



16 The Graph Of Y X2 6x 10 Cuts The Y Axis At A Gauthmath




Figure 2 The Curve Chas Equationy Y X2 5x 4 It Cut Gauthmath




If A Circle Passes Through A Point 1 2 And Cut The Circle X 2 Y
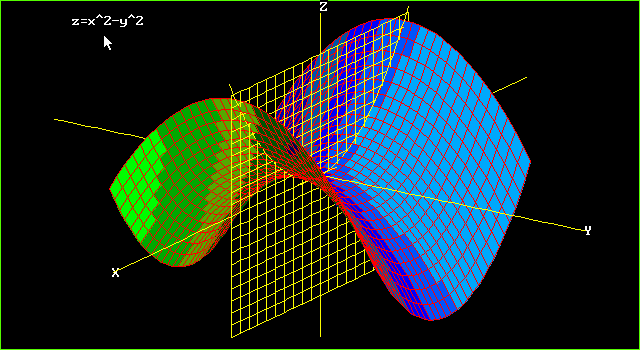



Graphs Of Surfaces Z F X Y Contour Curves Continuity And Limits




Prove That The Curves Y X 3 And Xy K Cut Each Other Orthogonal
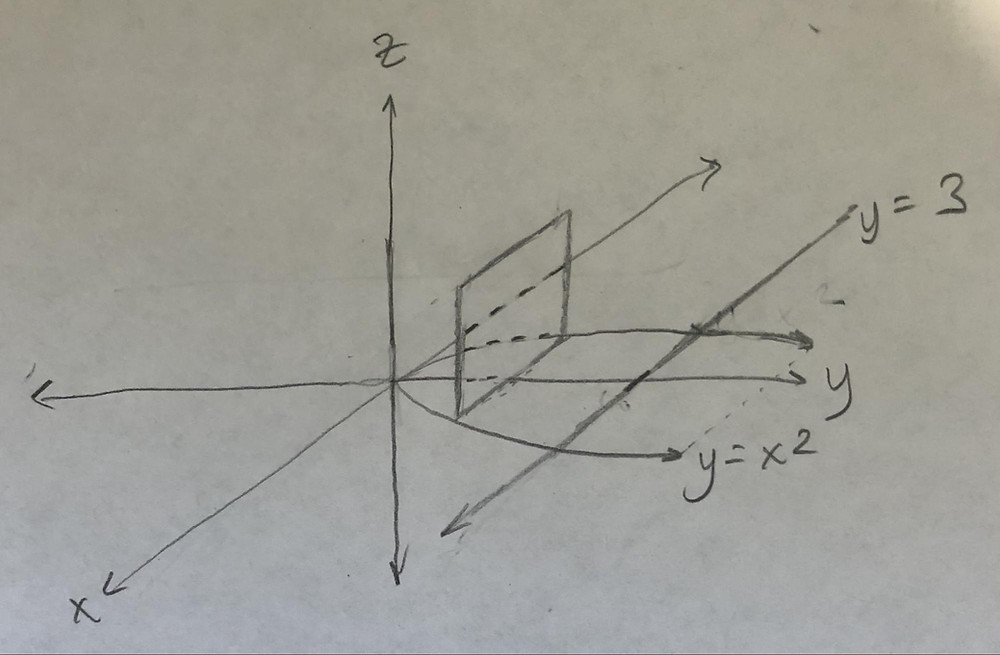



Volume Of A Solid Of Known Cross Section




Slowness Curves For Bulk Acoustic Waves In 128 O Yx Cut Linbo 3 Blue Download Scientific Diagram
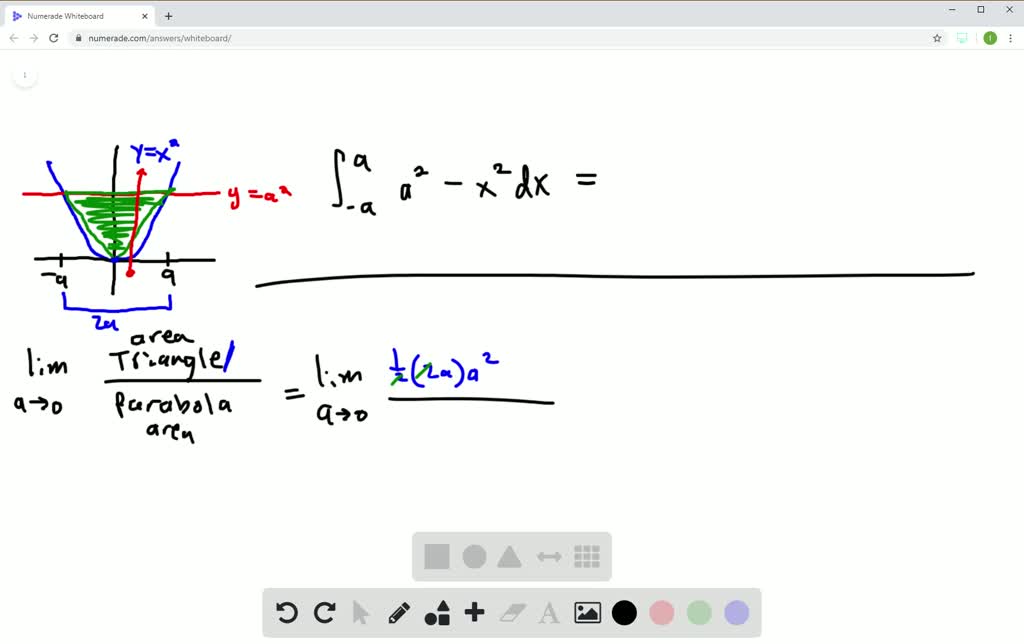



Solved The Figure Here Shows Triangle A O C Inscr
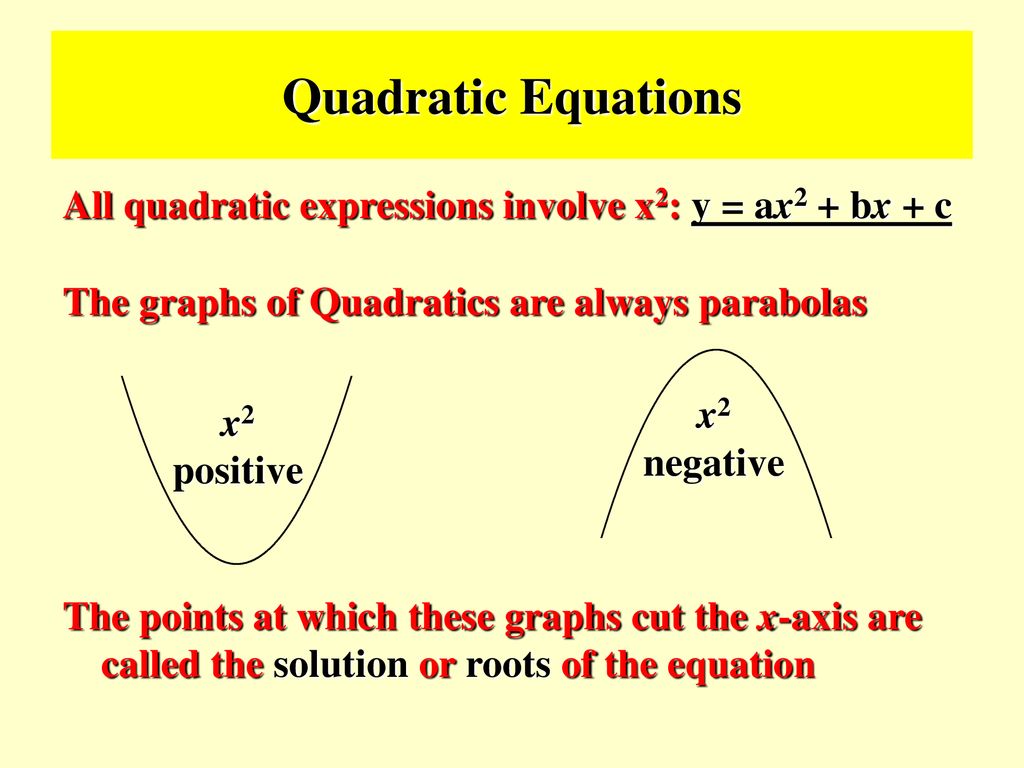



Quadratic Graphs Parabolas Ppt Download
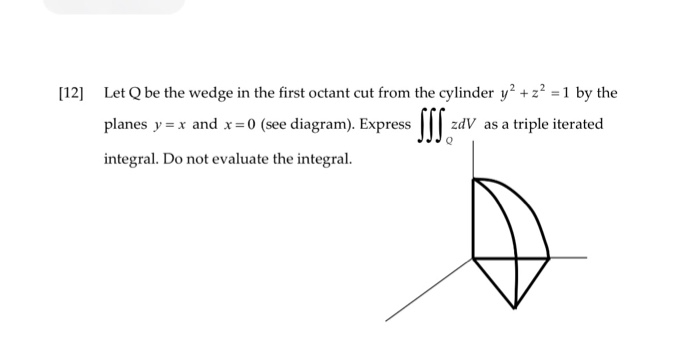



Let Q Be The Wedge In The First Octant Cut From The Chegg Com



Quadratics




A Comparative Study For Two Linbo3 Cuts Y Z And Y X In Detecting Bulk Acoustic Microwaves Using Probabilistic Neural Network Sciencedirect




Slowness Curve For 64 Yx Cut Linbo 3 Materials Constants From 16 Download Scientific Diagram



Find The Area Of The Portion Of The Surface Of The Sphere X 2 Y 2 Z 2 4x That Is Cut Off By A Nappe Of The Cone Y 2 Z 2 X 2 Mathematics Stack Exchange




Michael Cuts A Piece Of Wood For A Project The First Cut Is Shown And Can Be Represented By The Brainly Com
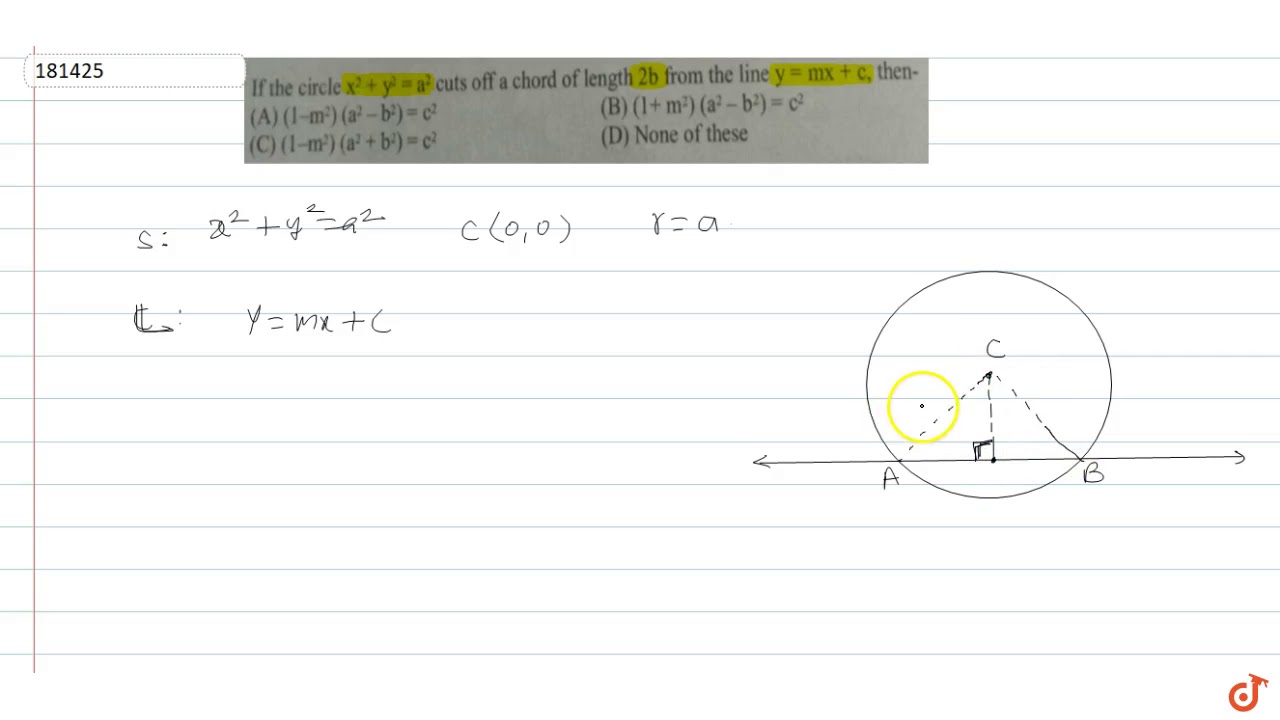



If The Circle X 2 Y 2 A 2 Cuts Off A Chord Of Length 2b From The Line Y Mx C Then Youtube
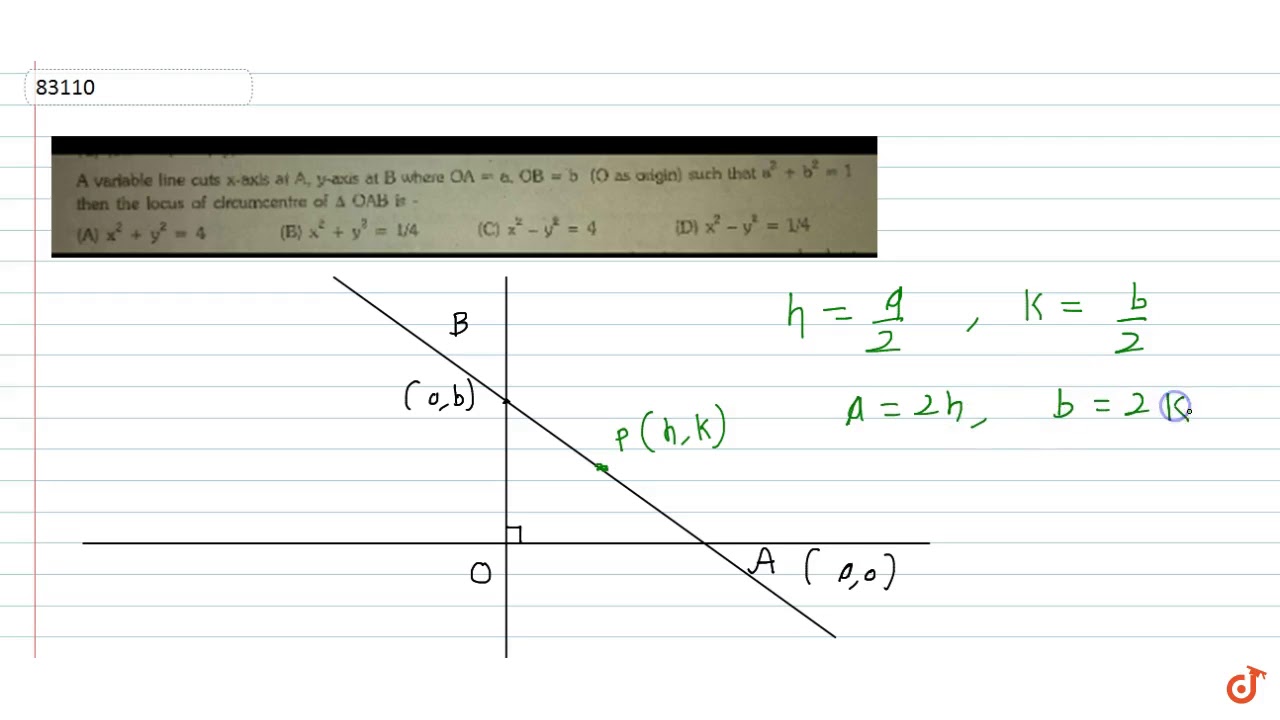



A Variable Line Cuts X Axis At A Y Axis At B Where Oa A Ob B O As Origin Such That Then Youtube
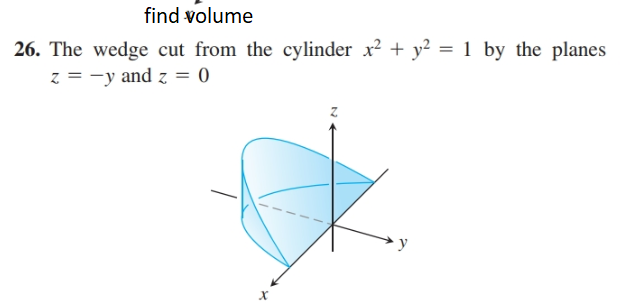



Answered Find Volume 26 The Wedge Cut From The Bartleby




2 C X Y X 4 Y 3 0 Is A C 1 Curve With Mfs C 0 Download Scientific Diagram




How To Find The Volume Of The First Octant Section Cut From The Region Inside The Cylinder Math X 2 Z 2 1 Math By The Planes Math Y 0 Z 0 X Y Math Quora



3



Graphing Systems Of Equations Cut And Paste Activity By Amusing Algebra



Ocw Mit Edu Resources Res 18 001 Calculus Online Textbook Spring 05 Textbook Mitres 18 001 Strang 8 Pdf



1



Quadratic Function Parabola



14 3 Partial Differentiation
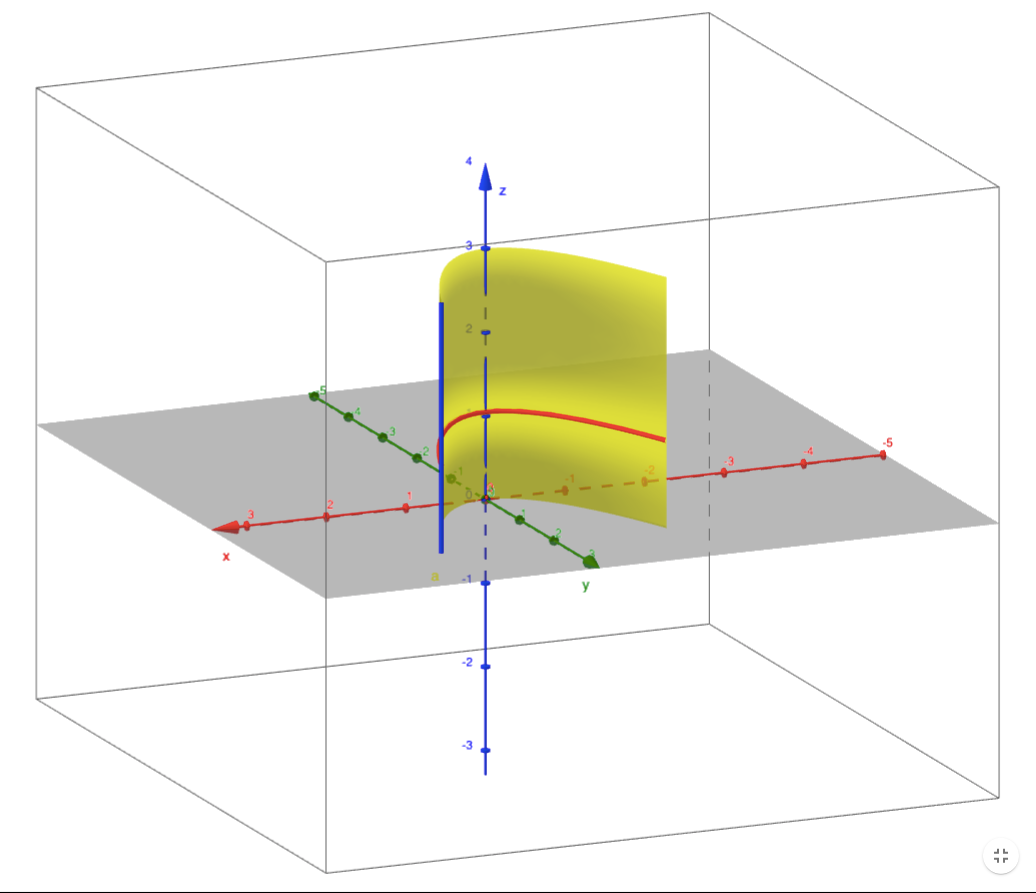



Find A Parametrization Of The Surface The Surface Cut From The Parabolic Cylinder Y X 2 By The Planes Z 0 Z 3 And Y 2 Homework Help And Answers Slader



Http Www3 Nd Edu Math Lecture notes 9 16 notes Pdf



Www Wssd K12 Pa Us Downloads Ap calculus exam prep assignment 4 probs key Pdf
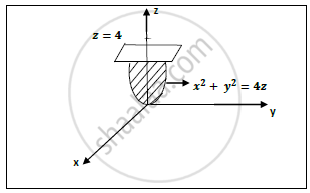



Find The Volume Of The Paraboloid X 2 Y 2 4 Z Cut Off By The Plane 𝒛 𝟒 Applied Mathematics 2 Shaalaa Com
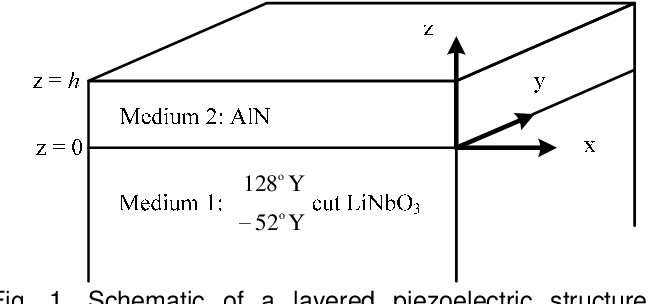



Pdf Propagation Characteristics Of Surface Acoustic Waves In Aln 128 Y X Linbo3 Structures Semantic Scholar




Answered Exercise 1 Find The Volume Of The Bartleby



Bailey Bridges




Calc3 1001 By James Bardo Issuu




Two Straight Lines Passing Through The Point A 3 2 Cut The Li




Calculus Volume Integral Mathematics Stack Exchange
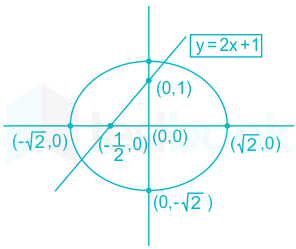



Solved The Length Of The Chord Cut Off By Y 2x 1 From The Circle X 2 Self Study 365



Http Onlinelibrary Wiley Com Doi 10 1002 Net Pdf
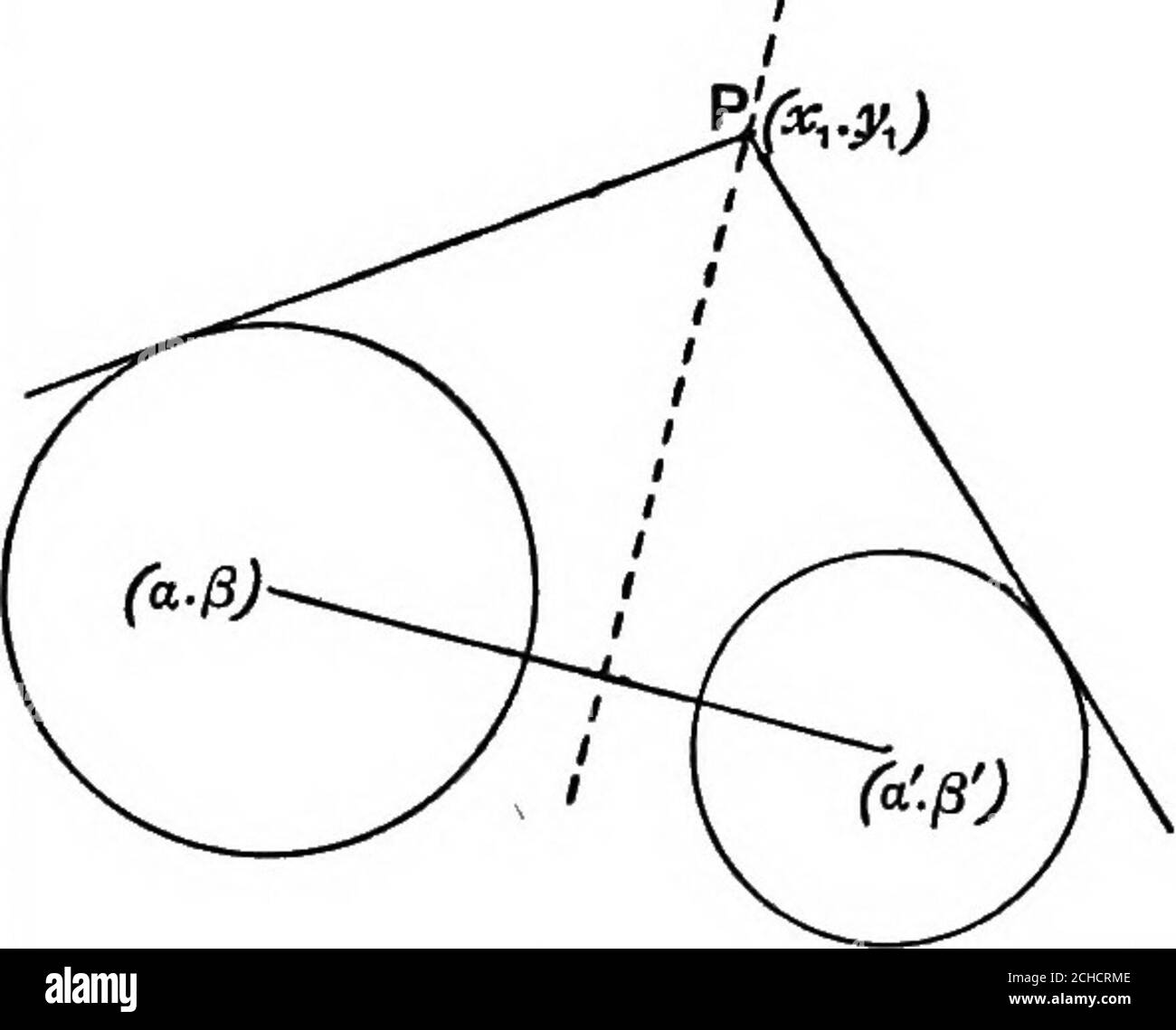



Algebraic Geometry A New Treatise On Analytical Conic Sections At The Point H H Bxg Vii A Orthogonal Circles 105 7 Three Circles Are Described Of Equal Radii R Having




Find The Surface Area Of The Surface Cut From The Paraboloid X 2 Y 2 Z 0 By The Plane Z Study Com
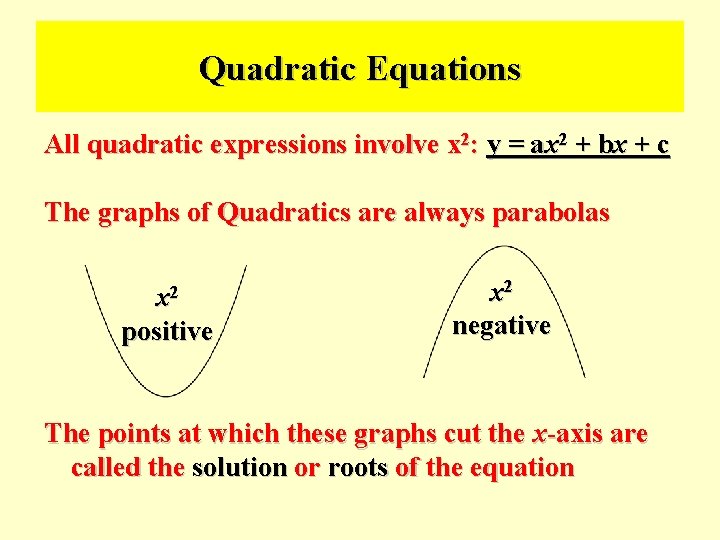



Quadratic Graphs Parabolas 1 To Draw A Quadratic




Let R Be The Region Bounded By Y X 2 And Y 4 Compute The Volume Of The Solid Formed By Revolving R About The Y Axis Study Com




Find The Smaller Area Cut From The Circle X 2 Y 2 25 By The Line X 3 N6
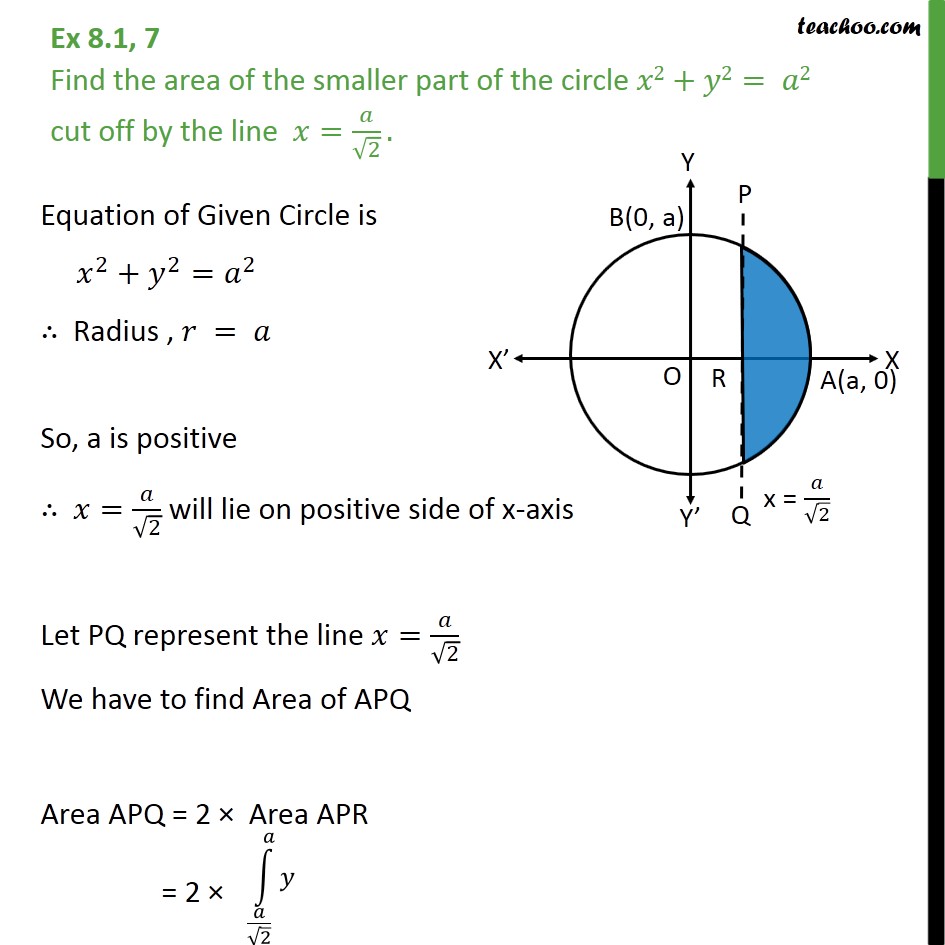



Ex 8 1 7 Find Area Of Smaller Part Of Circle X2 Y2 Cutoff



Absolute Value Graphs Horizontal And Vertical Shifts Cut And Paste Activity



Quadratic Function Parabola



Differential And Integral Calculus To The Circle X 4 2 Y 3 2 25 At The Point 7 1 7 Find The Equations Of The Tangents To The Hyperbola4x2 Gy2 36
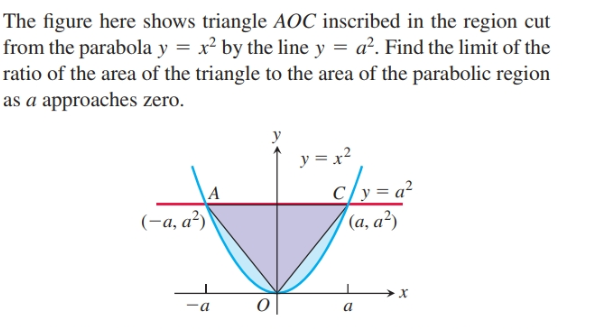



Answered The Figure Here Shows Triangle Aoc Bartleby
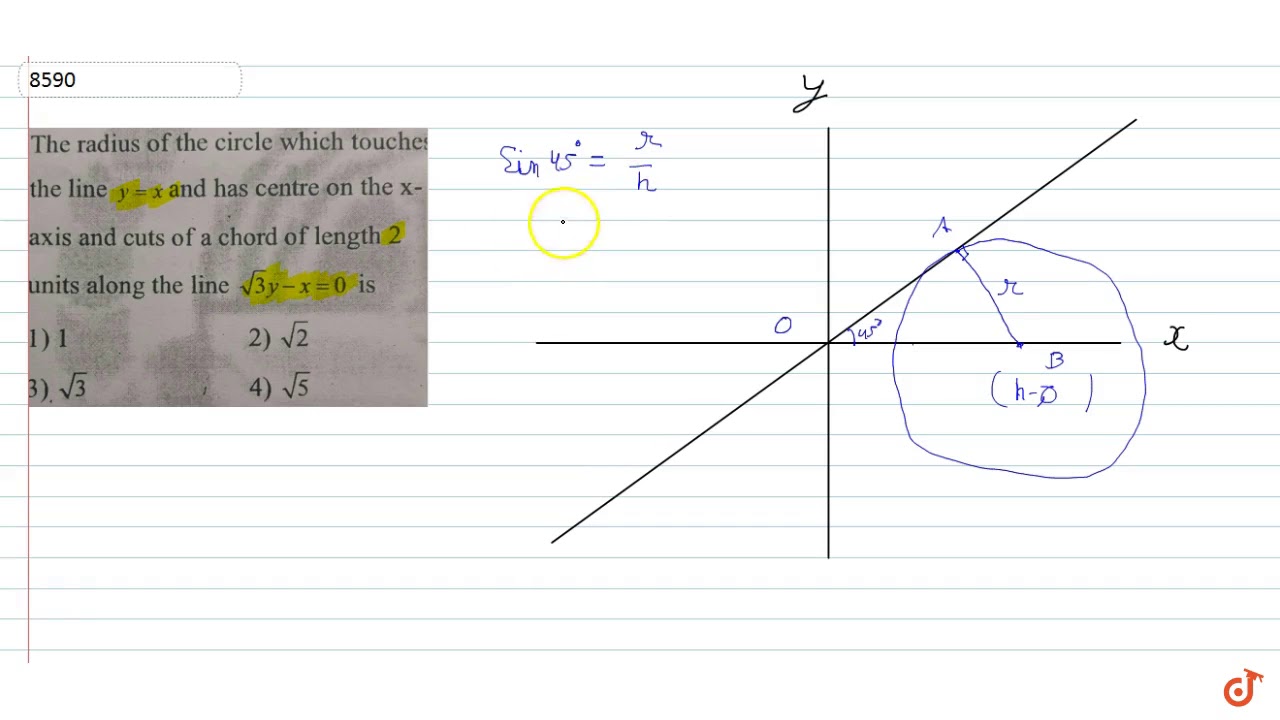



The Radius Of The Circle Touches The Line Y X And Has Centre On The X Axis And Cuts A Chord Of Youtube



A Xyz Coordinate System Rotated Around The X Axis And Y Cut Crystal Download Scientific Diagram
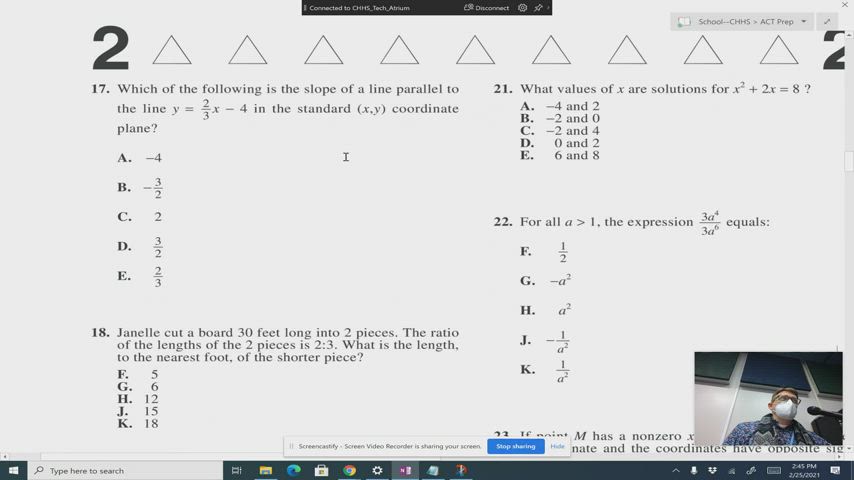



Act Math Review 2 February 25 21 Copper Hills High School
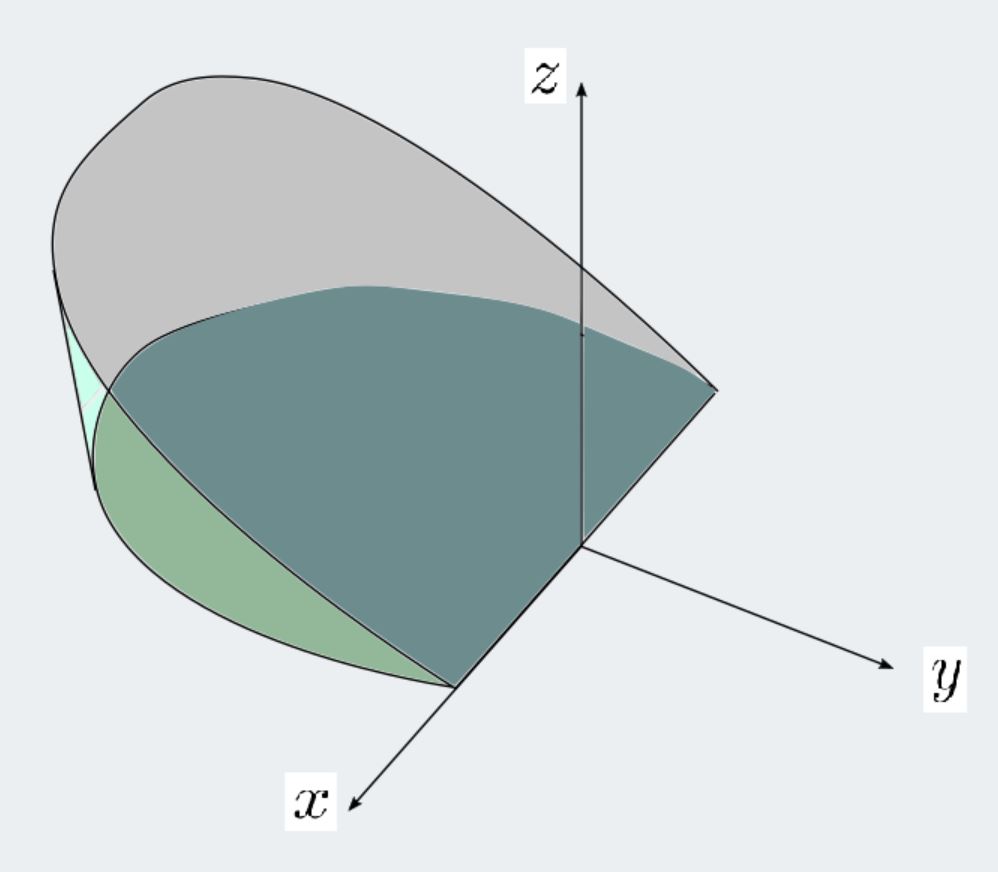



Hartleymath Triple Integrals




In What Ratio Does The Line Y X 2 0 Cut The Line Joining 3 1



0 件のコメント:
コメントを投稿